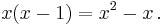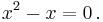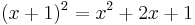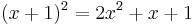Equation
An equation is a mathematical statement that asserts the equality of two expressions.[1] In modern notation, this is written by placing the expressions on either side of an equals sign (=), for example
asserts that x+3 is equal to 5. The = symbol was invented by Robert Recorde (1510–1558), who considered that nothing could be more equal than parallel straight lines with the same length.
Contents |
Knowns and unknowns
Equations often express relationships between given quantities, the knowns, and quantities yet to be determined, the unknowns. By convention, unknowns are denoted by letters at the end of the alphabet, x, y, z, w, …, while knowns are denoted by letters at the beginning, a, b, c, d, … . The process of expressing the unknowns in terms of the knowns is called solving the equation. In an equation with a single unknown, a value of that unknown for which the equation is true is called a solution or root of the equation. In a set simultaneous equations, or system of equations, multiple equations are given with multiple unknowns. A solution to the system is an assignment of values to all the unknowns so that all of the equations are true.
Types of equations
Equations can be classified according to the types of operations and quantities involved. Important types include:
- An algebraic equation is an equation involving only algebraic expressions in the unknowns. These are further classified by degree.
- A linear equation is algebraic equation of degree one.
- A polynomial equation is an equation in which a polynomial is set equal to another polynomial.
- A transcendental equation is an equation involving a transcendental function of one of its variables.
- A functional equation is an equation in which the unknowns are functions rather than simple quantities.
- A differential equation is an equation involving derivatives.
- An integral equation is an equation involving integrals.
- A Diophantine equation is an equation where the unknowns are required to be integers.
Identities
One use of equations is in mathematical identities, assertions that are true independent of the values of any variables contained within them. For example, for any given value of x it is true that
However, equations can also be correct for only certain values of the variables.[2] In this case, they can be solved to find the values that satisfy the equality. For example, consider the following.
The equation is true only for two values of x, the solutions of the equation. In this case, the solutions are 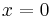 and
and 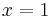 .
.
Many mathematicians[2] reserve the term equation exclusively for the second type, to signify an equality which is not an identity. The distinction between the two concepts can be subtle; for example,
is an identity, while
is an equation with solutions 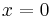 and
and 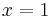 . Whether a statement is meant to be an identity or an equation can usually be determined from its context. In some cases, a distinction is made between the equality sign (
. Whether a statement is meant to be an identity or an equation can usually be determined from its context. In some cases, a distinction is made between the equality sign ( ) for an equation and the equivalence symbol (
) for an equation and the equivalence symbol ( ) for an identity.
) for an identity.
Letters from the beginning of the alphabet like a, b, c... often denote constants in the context of the discussion at hand, while letters from the end of the alphabet, like ...x, y, z, are usually reserved for the variables, a convention initiated by Descartes.
Properties
If an equation in algebra is known to be true, the following operations may be used to produce another true equation:
- Any real number can be added to both sides.
- Any real number can be subtracted from both sides.
- Any real number can be multiplied to both sides.
- Any non-zero real number can divide both sides.
- Some functions can be applied to both sides. Caution must be exercised to ensure that the operation does not cause missing or extraneous solutions. For example, the equation y*x=x has 2 solutions: y=1 and x=0. Dividing both sides by x "simplifies" the equation to y=1, but the second solution is lost.
The algebraic properties (1-4) imply that equality is a congruence relation for a field; in fact, it is essentially the only one.
The most well known system of numbers which allows all of these operations is the real numbers, which is an example of a field. However, if the equation were based on the natural numbers for example, some of these operations (like division and subtraction) may not be valid as negative numbers and non-whole numbers are not allowed. The integers are an example of an integral domain which does not allow all divisions as, again, whole numbers are needed. However, subtraction is allowed, and is the inverse operator in that system.
If a function that is not injective is applied to both sides of a true equation, then the resulting equation will still be true, but it may be less useful. Formally, one has an implication, not an equivalence, so the solution set may get larger. The functions implied in properties (1), (2), and (4) are always injective, as is (3) if we do not multiply by zero. Some generalized products, such as a dot product, are never injective.
More information at Equation solving.
See also
- Cubic equation
- Differential equation
- Diophantine equation
- Equation solving
- Formula editor
- Functional equation
- Indeterminate equation
- Inequality
- Inequation
- Integral equation
- Linear equation
- List of equations
- Quadratic equation
- Quartic equation
- Quintic equation
- Parametric equation
- Polynomial equation
- Scientific equations named after people
- Simultaneous equation
- Theory of equations
References
- ^ "Equation". Dictionary.com. Dictionary.com, LLC. http://dictionary.reference.com/browse/equation. Retrieved 2009-11-24.
- ^ a b Nahin, Paul J. (2006). Dr. Euler's fabulous formula: cures many mathematical ills. Princeton: Princeton University Press. p. 3. ISBN 0-691-11822-1.
External links
- Winplot: General Purpose plotter which can draw and animate 2D and 3D mathematical equations.
- Mathematical equation plotter: Plots 2D mathematical equations, computes integrals, and finds solutions online.
- Equation plotter: A web page for producing and downloading pdf or postscript plots of the solution sets to equations and inequations in two variables (x and y).
- EqWorld—contains information on solutions to many different classes of mathematical equations.
- EquationSolver: A webpage that can solve single equations and linear equation systems.